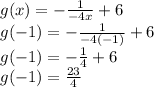Question

Given the following functions f(x) and g(x), evaluate (gºf)(-2). Enter answer.

f(x)=2x^2+2x-5
g(x)=-1/-4x+6​

Answer 1

the composition results in
`(23)/(4)`

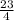
Explanation:

Assuming that what you typed for function g is correct (looks a bit peculiar, but we will use it as typed):

`g(x)=-(1)/(-4x) +6`

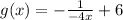
gof(-2) can be understood as: g(f(-2)), so what we need to do is to find the value for f(-2) using its given expression, and using then the obtained value as input for g(x):

`f(x)=2x^2+2x-5\\f(-2)=2\,(-2)^2+2\,(-2)-5\\f(-2)= 8-4-5\\f(-2)= -1`

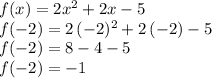
So, we now evaluate g(-1):

`g(x)=-(1)/(-4x) +6\\g(-1)= -(1)/(-4(-1)) +6\\g(-1)= -(1)/(4) +6\\g(-1)=(23)/(4)`