Answer:
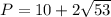 units
units
Explanation:
Given
Shape: Kite WXYZ
W (-3, 3), X (2, 3),
Y (4, -4), Z (-3, -2)
Required
Determine perimeter of the kite
First, we need to determine lengths of sides WX, XY, YZ and ZW using distance formula;

For WX:
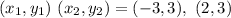

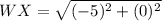

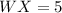
For XY:
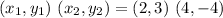
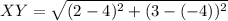
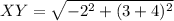
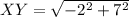
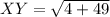
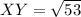
For YZ:

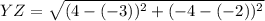
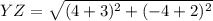

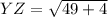
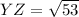
For ZW:


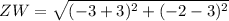
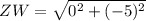
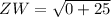
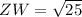
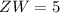
The Perimeter (P) is as follows:

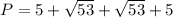
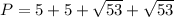
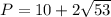 units
units