Question

For the function f(x) = 16x^3 - x, make a table of slopes of secant lines and make a conjecture about the slope of the tangent line at x = 1. Complete the table. (Round the final answer to three decimal places as needed. Round all intermediate values to four decimal places as needed.) An accurate conjecture for the slope of the tangent line at x = 1 is (Round to the nearest integer as needed.)

Answer 1

Complete Question

The complete question is shown on the first uploaded image

Answer:

Interval Slope of secant lines

[1,2] 113.000

[1, 1.5] 75.000

[1, 1.1 ] 51.960

[1, 1.01] 47.482

[1, 1.001] 47.048

Step-by-step explanation:

From the question we are told that

The function is
`f(x) =  16x^3 -x`

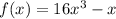
Now considering the intervals

For [1, 2]

The slopes of secant lines is mathematically represented as

`( f(2) - f(1))/(2-1)  =  (16(2)^3 - 2 - [16(1)^3 -1] )/(2-1 )  =  113.000`

For [1, 1.5]

The slopes of secant lines is mathematically represented as

`( f(1.5) - f(1))/(1.5-1)  =  (16(1.5)^3 - 1.5 - [16(1)^3 -1] )/(1.5-1 )  = 75.000`

For [1, 1.1]

The slopes of secant lines is mathematically represented as

`( f(1.1) - f(1))/(1.1-1)  =  (16(1.1)^3 - 1.1 - [16(1)^3 -1] )/(1.1-1 )  = 51.960`

For [1, 1.01]

The slopes of secant lines is mathematically represented as

`( f(1.01) - f(1))/(1.01-1)  =  (16(1.01)^3 - 1.01 - [16(1)^3 -1] )/(1.01-1 )  =47.482`

For [1, 1.001]

The slopes of secant lines is mathematically represented as

`( f(1.001) - f(1))/(1.001-1)  =  (16(1.01)^3 - 1.001 - [16(1)^3 -1] )/(1.001-1 )  =47.048`