Question

Write the equation of the line that goes through the point

(7, 10) and is parallel to the line y = 2x + 5

Answer 1

Use point slope equation.

Since parallel, the slope is the same. Then jsut plug in the numbers to the formula (u can google this)

y - 10 = 2 (x - 7)

^^this is point slope form

for slope intercept:

y = 2x - 4

Answer 2

`y=2x-4`

Explanation:

So we want to write an equation of a line that goes through the point (7,10) and is parallel the the line y=2x+5.

First, we can determine the slope of our new line. Parallel lines always have the same slope. In other words, the slope of our new line must also be 2.

Now, we can use the point-slope form to find our equation:

`y-y_1=m(x-x_1)`

Let's let (7,10) be x₁ and y₁. Let's also substitute 2 for m. Thus:

`y-10=2(x-7)`

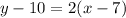
Distribute:

`y-10=2x-14`

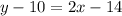
Add 10 to both sides:

`y=2x-4`

And we're done!