Answer:
Not always but sometimes
Explanation:
The product of two irrational numbers is sometimes and not always an irrational number.
So, Let us take two Example:
Example # 1:
Let the two irrational numbers be

So, Multiplying these will give us
=>

=>
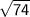
Which is an irrational number.
Example # 2:
Let the two irrational numbers be

So, Multiplying them would give us:
=>

=>

=> 2
Which is a rational number.
This means that the product of two irrational numbers is not always an irrational number but sometimes an irrational number.