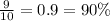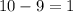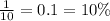Answer:
65%
Explanation:
We're looking for how big a part of the original price the reduced price is.
This can be done using division.
E.g., if the reduced price was $1, and the original price was $10, we can find how big of a part $1 is of $10 by dividing them.
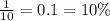
The reduced price is 10% of the original price. Remember that 0.1 in decimal is 10%, same as how 1.0 is 100%, and 0.3 is 30%.
Let's try this using our dresses. The reduced price was $39, and the original price was $60.
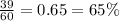
The dresses were sold at 65% of the original price.
Note, this does NOT mean that they were sold at a 65% discount. To find that, we'd have to find out how big the discount was. Then, we'd divide it by the original price.
For example, if the original price was $10, and the reduced price is $9, then the reduced price is 90% of the original price, but it's at a 10% discount.