Question

Find the solutions of the quadratic equation 5x^2 +3x+1=0

Answer 1

The answer is

`x = - (3)/(10) + ( √(11) )/(10) \: i \\ \\ \: OR \\ \\ x = - ( 3)/(10) - ( √(11) )/(10) \: i`

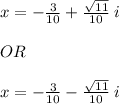
Explanation:

5x² + 3x + 1 = 0

Using the quadratic formula

`x = \frac{ - b\pm \sqrt{ {b}^(2) - 4ac } }{2a}`

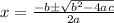
From the question

a = 5 , b = 3 , c = 1

Substitute the values into the above formula and solve

That's

`x = \frac{ - 3\pm \sqrt{ {3}^(2) - 4(5)(1) } }{2(5)} \\ x = ( - 3\pm √(9 - 20) )/(10) \\ x = ( - 3\pm √( - 11) )/(10) \\ x = ( - 3\pm √(11 ) \: i)/(10) \\ x = ( - 3 + √(11) \: i)/(10) \: \: or \: \: \: x = ( - 3 - √(11) \: i)/(10)`

Separate the real and imaginary parts

We have the final answer as

`x = - (3)/(10) + ( √(11) )/(10) \: i \\ \\ x = - ( 3)/(10) - ( √(11) )/(10) \: i`

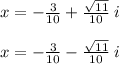
The equation has complex roots

Hope this helps you