A product has a production cost function C(x) =165x + 3630 and a revenue function R(x)= 220x. Find and analyze the break-even quantity, then find the profit function.
Answer:
the break-even quantity = 66
the profit function = 55x - 3630
Explanation:
Given that:
C(x) = 165x + 3630
R(x) = 220 x
The Break-even quantity is C(x) = R(x)
∴
165x + 3630 = 220x
collecting the like terms, we have:
3630 = 220 x - 165 x
3630 = 55 x
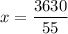
x = 66
Thus, the break-even quantity = 66
The profit function = R(x) - C(x)
The profit function = 220x - 3630 - 165x
By rearrangement
The profit function = 220x - 165x - 3630
The profit function = 55x - 3630