Answer:
0.1π rad
Explanation:
The question is incomplete. Here is the complete question.
A circle has a radius of 19m . Find the radian measure of the central angle θ that intercepts an arc of length 5m. Do not round any intermediate computations, and round your answer to the nearest tenth.
The formula for calculating the length of an arc
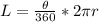
θ is the central angle
r is the radius of the circle = 19m
L is the length of an arc = 5m
Substitute the given values into the formula and get the central angle θ
5 = θ/360 * 2(22/7)*19
5 = θ/2π * 119.4285714
θ/2π = 5/119.4285714
θ/2π = 0.041866
θ = 2π*0.041866
θ = 0.083732π
θ = 0.1π rad (to the nearest tenth)
Hence the radian measure of the central angle that intercepts an arc of length to the nearest tenth is 0.1π rad