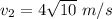Complete Question
A small metal sphere, carrying a net charge q1=−2μC, is held in a stationary position by insulating supports. A second small metal sphere, with a net charge of q2= -8μC and mass 1.50g, is projected toward q1. When the two spheres are 0.80m apart, q2 is moving toward q1 with speed 20ms−1. Assume that the two spheres can be treated as point charges. You can ignore the force of gravity.The speed of q2 when the spheres are 0.400m apart is.
Answer:
The value
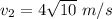
Step-by-step explanation:
From the question we are told that
The charge on the first sphere is
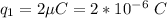
The charge on the second sphere is
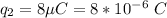
The mass of the second charge is
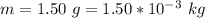
The distance apart is
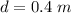
The speed of the second sphere is
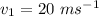
Generally the total energy possessed by when
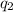 and
and
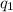 are separated by
are separated by
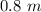 is mathematically represented
is mathematically represented
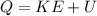
Here KE is the kinetic energy which is mathematically represented as

substituting value
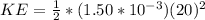
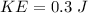
And U is the potential energy which is mathematically represented as
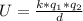
substituting values
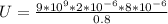
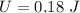
So

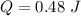
Generally the total energy possessed by when
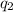 and
and
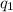 are separated by
are separated by
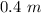 is mathematically represented
is mathematically represented
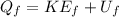
Here
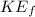 is the kinetic energy which is mathematically represented as
is the kinetic energy which is mathematically represented as
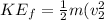
substituting value
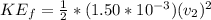
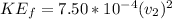
And
 is the potential energy which is mathematically represented as
is the potential energy which is mathematically represented as
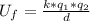
substituting values
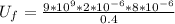
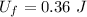
From the law of energy conservation
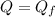
So