Answer:
62.93%
Explanation:
Z score is a score used in statistics to measure by how many standard deviations that the raw score is above or below the mean. A positive z score means the raw score is above the mean and a negative z score means the raw score is below the mean. The z score is given as:

Given that:
Mean (μ) = 30.05 inches, standard deviation (σ) = 0.3 inches
For x > 29.95
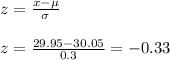
From the normal distribution table, P(x > 29.95) = P(z > -0.33) = 1 - P(z < 0.33) = 1 - 0.3707 = 0.6293 = 62.93%