Question

У=x+5 3x+y=25 substitution

Answer 1

(5,10)

Explanation:

So we have the system of equations:

`y=x+5\\3x+y=25`

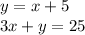
To solve, we can use substitution.

Substitute the first equation into the second:

`3x+y=25\\3x+(x+5)=25`

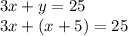
Combine like terms:

`4x+5=25`

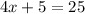
Subtract 5:

`4x=20`

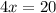
Divide by 4:

`x=5`

Now, substitute this into the first equation:

`y=x+5\\y=5+5`

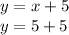
Add:

`y=10`

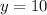
So, our answer is (5,10)

And we are done :)