Question

) A company determines that its marginal revenue per day is given by R'(t) = 100et , R(0) = 0, where R(t) = the revenue, in dollars, on the tth day. The company's marginal cost per day is given by C'(t) = 140 - 0.3t, C(0) = 0, where C(t) = the cost, in dollars, on the tth day. Find the total profit from t = 0 to t = 5 (the first 5 days). Round to the nearest dollar. Note: P(T) = R(T) - C(T) = T 0 ∫ [R'(t) - C'(t)] dt.

Answer 1

The answer is below

Step-by-step explanation:

The marginal revenue R'(t) =
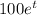`100e^t` and the marginal cost C'(t) = 140 - 0.3t.

The total profit is the difference between the total revenue and total cost of a product, it is given by:

Profit = Revenue - Cost

P(T) = R(T) - C(T)

P(T) = ∫ R'(T) - C'(T)

Hence the total profit from 0 to 5 days is given as

`P(T) = \int\limits^0_5 {(R'(T)-C'(T))} \, dt= \int\limits^0_5 {(100e^t-(140-0.3t))} \, dt\\ \\P(T)= \int\limits^0_5 {(100e^t-140+0.3t))} \, dt\\\\P(T)= \int\limits^0_5 {100e^t} \, dt- \int\limits^0_5 {140} \, dt+ \int\limits^0_5 {0.3t} \, dt\\\\P(T)=100\int\limits^0_5 {e^t} \, dt- 140\int\limits^0_5 {1} \, dt+0.3 \int\limits^0_5 {t} \, dt\\\\P(T)=100[e^t]_0^5-140[t]_0^5+0.3[(t^2)/(2) ]_0^5\\\\P(T)=100(147.41)-140(5)+0.3(12.5)=14741-700+3.75\\\\P(T)=14045`