Question

-2|5x-1|-3=11 and -2|5x-1|-3=-11

Answer 1

Answer : The values of x are
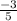`(-3)/(5)` and 1.

Step-by-step explanation :

The given equation is:

`-2|5x-1|-3=-11`

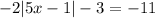
The term -3 is moved or added to the right hand side.

`-2|5x-1|=-11+3`

`-2|5x-1|=-8`

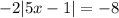
The term -2 is divided to the right hand side.

`|5x-1|=(-8)/(-2)`

`|5x-1|=4`

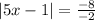
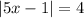
Clear the absolute-value bars by splitting the equation into two cases. One for the positive case and the other for the negative case.

Negative case :
`-(5x-1)=4`

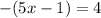
Positive case :
`(5x-1)=4`

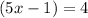
Now solve both the cases.

Negative case :
`-(5x-1)=4`

`-5x+1=4`

`-5x=4-1`

`-5x=3`

`x=(-3)/(5)`

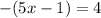
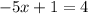
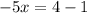
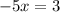
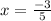
and,

Positive case :
`(5x-1)=4`

`5x=4+1`

`5x=5`

`x=1`

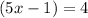
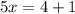
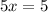
Therefore, the values of x are
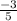`(-3)/(5)` and 1.