Question

After a shipwreck, 120 rats manage to swim from the wreckage to a deserted island. The rat population on the island grows exponentially, and after 15 months, there are 280 rats on the island.

A. Find a function that models the population t months after the arrival of the rats.
B. What will the population be 3 years after the shipwreck?
C. When will the population reach 2000 rats?

Answer 1

a.
`X(T) = 120 (1.058)^T`

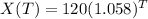
b. Population after 3 years is 142

c. 50 years

Explanation:

Given

Type of growth: Exponential

Initial number of rats = 120

Number of rats (15months) = 280

Solving (a)

Since the growth type is exponential, we make use of the following exponential progression

`X_T = X_0 (1 + R)^T`

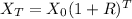
Where Xo is the initial population;

Xo = 125

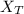`X_T` is the current population at T month

So;

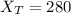`X_T = 280`; when
`T = 15`

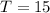
Substitute these values in the above formula

`280 =120 * (1 + R)^(15)`

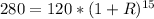
Divide both sides by 120

`(280)/(120) =(1 + R)^(15)`

`2.3333 =(1 + R)^(15)`

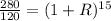
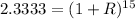
Take 15th root of both sides

`\sqrt[15]{2.3333} =1 + R`

`1.05811235561 = 1 + R`

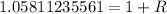
Subtract 1 from both sides

`R = 1.05811235561 - 1`

`R = 0.05811235561`

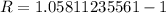
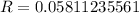
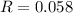`R = 0.058` (Approximated)

Plug in values of R and Xo in
`X_T = X_0 (1 + R)^T`

`X_T = 120 (1 + 0.058)^T`

`X_T = 120 (1.058)^T`

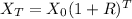
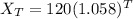
Write as a function

`X(T) = 120 (1.058)^T`

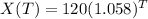
Hence, the function is
`X(T) = 120 (1.058)^T`

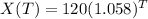
Solving (b):

Population after 3 years

In this case, T = 3

So:

`X(T) = 120 (1.058)^T`

`X(3) = 120 (1.058)^3`

`X(3) = 120 * 1.18466445254`

`X(3) = 142.159734305`

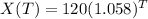
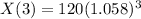
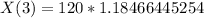
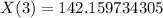
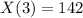`X(3) = 142` (Approximated)

Solving (c): When population will reach 2000

Here: X(T) = 2000

So:

So:

`2000 = 120 (1.058)^T`

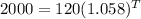
Divide both sides by 120

`(2000)/(120) = 1.058^T`

`16.667 = 1.058^T`

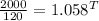
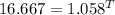
Take Log of both sides

`Log(16.667) = Log(1.058^T)`

Apply law of logarithm

`Log(16.667) = TLog(1.058)`

Divide both sides by Log(1.058)

`T = (Log(16.667))/(Log(1.058))`

`T = 49.9009236926`

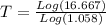
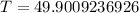
Approximate

`T = 50\ years`