Answer:
It is given that the volume of a cone =
 cubic units
cubic units
Volume of cone with radius 'r' and height 'h' =
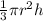
Equating the given volumes, we get
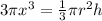
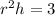 ×
×
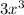
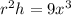
It is given that the height is 'x' units.
Therefore,
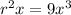
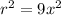
Therefore,
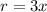
So, the expression '
 ' represents the radius of the cone's base in units.
' represents the radius of the cone's base in units.