Answer:
The standard error is 0.033.
Explanation:
We are given that Sukie interviewed 125 employees at her company and discovered that 21 of them planned to take an extended vacation next year.
Let
 = proportion of employees who planned to take an extended vacation next year
= proportion of employees who planned to take an extended vacation next year
 =
=
 =
=
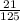 = 0.168
= 0.168
n = number of employees at her company = 125
Now, the standard error is calculated by the following formula;
Standard error, S.E. =

=
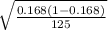
=
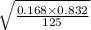 = 0.033
= 0.033
Hence, the standard error is 0.033.