Answer:
a. y(t) = - 1/2gt² + y₀ b.
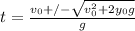
Explanation:
Let the quadratic function y(t) = v₀t - 1/2gt² + y₀ represent the quadratic function that models the height above the ground of the projectile.
a. Maximum Height
At maximum height, the velocity, v₀ = 0, so substituting v₀ = 0 into the equation, we have
y(t) = v₀t - 1/2gt² + y₀
y(t) = 0 × t - 1/2gt² + y₀
y(t) = 0 - 1/2gt² + y₀
y(t) = - 1/2gt² + y₀
b. Time when the projectile reaches the ground
The time when the projectile reaches the ground is gotten when y(t) = 0, So
y(t) = v₀t - 1/2gt² + y₀
0 = v₀t - 1/2gt² + y₀
re-arranging, we have
1/2gt² - v₀t - y₀ = 0
Using the quadratic formula,
