Answer:
The P-value is between 2.5% and 5% from the t-table.
Explanation:
We are given that a random sample of 16 students selected from the student body of a large university had an average age of 25 years and a standard deviation of 2 years.
Let
 = true average age of all the students at the university.
= true average age of all the students at the university.
So, Null Hypothesis,
 :
:
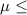 24 years {means that the average age of all the students at the university is less than or equal to 24}
24 years {means that the average age of all the students at the university is less than or equal to 24}
Alternate Hypothesis,
 :
:
 > 24 years {means that the average age of all the students at the university is significantly more than 24}
> 24 years {means that the average age of all the students at the university is significantly more than 24}
The test statistics that will be used here is One-sample t-test statistics because we don't know about the population standard deviation;
T.S. =
 ~
~

where,
 = sample average age = 25 years
= sample average age = 25 years
s = sample standard deviation = 2 years
n = sample of students = 16
So, the test statistics =
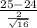 ~
~
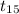
= 2
The value of t-test statistics is 2.
Also, the P-value of test-statistics is given by;
P-value = P(
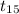 > 2) = 0.034 {from the t-table}
> 2) = 0.034 {from the t-table}
The P-value is between 2.5% and 5% from the t-table.