Question

Help me with this.. thanks

Answer 1

Attachment 1 :- x = 3/2

Attachment 2 :- m + r = 154°

Explanation:

Attachment 1 :-

`9^(^2^-^x^) = 3\\=> 3^2^(^2^-^x^) = 3\\`

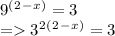
As the base 3 is same on both the sides , cancel the base 3 from both left & right side of eqn. After that we will get:-

`2(2 - x) = 1\\=> 2 - x =(1)/(2) \\=> x = 2 - (1)/(2) = (3)/(2)`

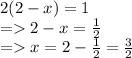
Attachment 2 :-

PQR is a straight line . It's given that m + n = 110°

But we know that m + n + r = 180° ..............eqn.1

So substituting the value of m+n in eqn.1 gives :-

m+n+r = 180°

=> r + 110° = 180°

=> r = 180° - 110°

= 70°

It's also given that n+r = 96°

So putting the value of r in the above gives ,

n+r = 96°

=> n + 70° =96°

=> n = 96° - 70°

= 26°

Putting the value of n in eqn.1 gives

m + n = 110°

=> m + 26° = 110°

=> m = 110° - 26°

= 84°

So m + r = 84° + 70° = 154°