1 Answer

Kirill Shlenskiy · Answer 1 · 2021-12-05T22:37:43+0000

Answer:

A

Explanation:

So we have the function:

$f(x)=y=\sqrt[3]{x-2}+8$

To find the inverse of the function, we can flip x and y and then solve for y. Therefore:

$x=\sqrt[3]{y-2}+8$

Solve for y to find our inverse.

Subtract 8 from both sides:

$x-8=\sqrt[3]{y-2}$

Cube each side. The right cancels:

(x-8)^3=y-2

Add 2 to both sides:

y=(x-8)^3+2

Therefore, our inverse is:

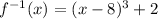
f^(-1)(x)=(x-8)^3+2

The answer is A

Please log in or register to add a comment.