Question

Samuel currently has an account balance of $3,439.96. He opened the account five years ago with a deposit of $3,104.31. If the interest compounds quarterly, what is the interest rate on the account?

Answer 1

The interest rate on the account is 2.1%

Explanation:

Compound interest is one that is added to the initial capital and on which new interests are generated. Money, in this case, has a multiplier effect because interest produces new interest. In other words, the initial capital increases in each period because the interests add up.

To calculate the final capital, the initial capital is multiplied by one plus the interest, raised to the number of periods:

`A=P*(1+(r)/(n)) ^(n*t)`

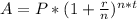
where

• A = Accumulated amount (principal + interest)
• P = Principal amount
• r = the interest rate
• n = the number of times the interest is compounded per year
• t = Time Involved in years

In this case:

• A = $3,439.96
• P = $3,104.31
• r = ?
• n = 4
• t = 5

Replacing, you get:

`3,439.96=3,104.31*(1+(r)/(4)) ^(4*5)`

Now, solving:

`(3,439.96)/(3,104.31) =(1+(r)/(4)) ^(20)`

`\sqrt[20]{1.1081} =(1+(r)/(4))`

`1.00514 =1+(r)/(4)`

`1.00514 -1=(r)/(4)`

`0.00514=(r)/(4)`

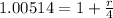
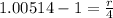
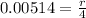
r=0.0051*4

r= 0.02056

In percentage it is expressed as: r= 2.056 %≅ 2.1 %

The interest rate on the account is 2.1%