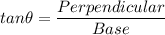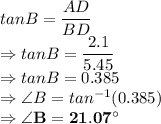Question

A gable roof (isosceles triangle-shaped) has a vertical height of 2.1 metres and the ceiling is 10.9 meters from one side to the other. Find the pitch (angle) of the roof.

Answer 1

`\bold{21.07^\circ}`

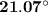
Explanation:

The given values can be mapped to an isosceles
`\triangle ABC`.

Side AB = AC

Vertical height, AD = 2.1 m

The distance between one side to the other side of ceiling = 10.9 m

To find:

Pitch (Angle of the roof ) = ?

i.e.
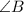`\angle B` or
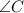`\angle C` = ? (because it is isosceles triangle, so both will be equal)

Solution:

As
`\triangle ABC` is isosceles, so vertical height will divide the side BC in two equal parts

i.e.
`BD = DC = (1)/(2) BC`

`\therefore BD = (10.9)/(2) = 5.45 m`

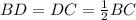
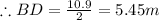
In
`\triangle ABD`, let us use tangent trigonometric property.

`tan\theta = (Perpendicular)/(Base)`

`tanB = (AD)/(BD)\\\Rightarrow tanB = (2.1)/(5.45)\\\Rightarrow tanB = 0.385\\\Rightarrow \angle B = tan^(-1)( 0.385)\\\Rightarrow \bold{\angle B = 21.07^\circ}`