Question

Part 6: please assist me with these problems​

Answer 1

Answer: 12) 34° 13) 90°

Explanation:

`\text{Law of Sines:}\quad (\sin A)/(a)=(\sin B)/(b)=(\sin C)/(c)`

12) Given: a = 10.2, b = 6.8, A = 122°

`(\sin 122^o)/(10.2)=(\sin B)/(6.8)\\\\\\(6.8\sin 122^o)/(10.2)=\sin B\\\\\\\sin^(-1)\bigg((6.8\sin 122^o)/(10.2)\bigg)=B\\\\\\34.4^o=B`

*****************************************************************************

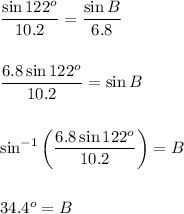
Law of Cosines: a² = b² + c² - 2bc · cos A

Note: The letters can be swapped

13) Given: a = 3, b = 4, c = 5, C = ???

3² = 4² + 5² - 2(4)(5) · cos C

9 = 16 + 25 - 40 cos C

9 = 41 - 40 cos C

-32 = -40 cos C

0.8 = cos C

90° = C