Question

Fill in the blank with a constant, so that the resulting quadratic is the square of a binomial. \[x^2 + \dfrac{4}{5}x + \underline{~~~~}\]

Answer 1

4/25

Explanation:

Suppose the quadratic is the square of the binomial $x+c.$ We have $(x+c)^2 = x^2+2cx + c^2.$ Comparing the linear term of $x^2 + 2cx+c^2$ to the linear term of $x^2 + \frac{4}{5}x + \underline{~~~~},$ we have $2c = \dfrac{4}{5},$ so $c = \dfrac{2}{5}.$ Therefore, the constant term is

\[c^2 = \left(\dfrac{2}{5}\right)^2 = \boxed{\frac{4}{25}}.\]

Answer 2

4/25

Explanation:

Given the incomplete quadratic equation
`\[x^2 + (4)/(5)x + \underline{~~~~}\]`, we are to complete it so that the result will be the square of a binomial. To do that, we will use the completing the square method.

Completing the square method is a way of completing a quadratic equation by solving for a constant to add that will make the quadratic equation a perfect square of a binomial.

To get the constant term using the method, we will take the square of the alf of the coefficient of x in the equation given.

Coefficient of x = 4/5

half of the coefficient of x = 1/2(4/5)

half of the coefficient of x = 4/10 = 2/5

Square of the half of the coefficient of x = (2/5)²

Square of the half of the coefficient of x = 4/25

Hence the constant that we will use to complete the equation to make if a square of a binomial is 4/25

The equation will become:

`= \[x^2 + (4)/(5)x +(4)/(25) \\\\= \[x^2 + (4)/(5)x +((2)/(5))^2\\\\= (x +(2)/(5))^2`

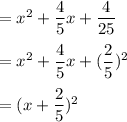
Hence the constant value that will make the resulting quadratic the square of a binomial is 4/25