Question

Earth rotates on an axis through its poles. The distance from the axis to a location on Earth at 40 degrees north latitude is about 3033.5 miles. Therefore a location on Earth at 40 degree north latitude is spinning on a circle of radius 3033.5 miles. Compute the linear speed on the surface of the Earth at 40 degrees north latitude.

Answer 1

Answer: v = 793.77 mph

Explanation: Linear speed of a circular movement is calculated by using the formula:

v = ω.r

where

ω is angular velocity

r is radius of the path

Angular velocity is the rate of change of the angular position of an object with respect of time, i.e., is how fast an object changes its angular position with time:

`\omega = (d \theta)/(dt)`

`\omega = (2\pi)/(time)`

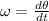
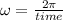
The Earth takes 24 hours to complete a rotation, then angular velocity:

`\omega = (2\pi)/(24)`

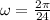
`\omega =` 0.262 rad/h

At a location 40° north, radius is 3033.5 miles, so:

v = 0.262*3033.5

v = 793.77

At latitude of 40°north, linear speed is 793.77mph.