Question

If the 11th term of a geometric sequence is 32 times larger than the 6th term, then

what is the common ratio of the sequence

Answer 1

r = 2

Explanation:

nth term of a geometric sequence =
`ar^(n - 1)`.

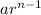Where,

a = first term, r = common ratio,

We are given that, the 11th term is 32 times larger than the 6th term of the geometric sequence. Thus:

the 6th term can be expressed as
`ar^(n - 1) = ar^(6 - 1)`

`a_6 = ar^(5)`

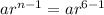
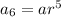
The 11th term is expressed as
`a_11 = ar^(10)`

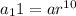
Since the 11th term is 32 times larger than the 6th term, therefore, the following equation can be derived to find the common ratio, r, of the sequence:

`a_11 = 32*a_6`

`a_11 = ar^(10)`

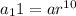
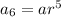`a_6 = ar^(5)`, therefore:

`ar^(10) = 32*ar^(5)`

Divide both sides by ar⁵

`(ar^(10))/(ar^5) = (32*ar^(5))/(ar^5)`

`r^5 = 32`

`r^5 = 2^5`

`r = 2`

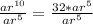
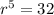
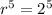
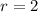
Common ratio = 2