Question

Use the First Derivative Test to determine whether the critical point is a local min or max (or neither). (Enter your answers as a comma-separated list. If an answer does not exist, enter DNE.)

f(x)=(ln(2x)/8x)-7 (x>0)


Also determine the intervals on which the function is increasing or decreasing.

Answer 1

Explanation:

Let be
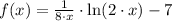`f(x) = (1)/(8\cdot x)\cdot \ln(2\cdot x) -7`, the first and second derivatives of the function are, respectively:

`f'(x) = (\left((2)/(2\cdot x)\right)\cdot 8\cdot x-8\cdot \ln (2\cdot x) )/(64\cdot x^(2))`

`f'(x) = (8-8\cdot \ln (2\cdot x))/(64\cdot x^(2))`

`f'(x) = (1-\ln(2\cdot x))/(8\cdot x^(2))`

`f''(x) = (\left(-(2)/(2\cdot x) \right)\cdot (8\cdot x^(2))-[1-\ln(2\cdot x)]\cdot (16\cdot x))/(64\cdot x^(4))`

`f''(x) = (-8\cdot x-16\cdot x+16\cdot x\cdot \ln (2\cdot x))/(64\cdot x^(4))`

`f''(x) = (-24\cdot x+16\cdot x \cdot \ln (2\cdot x))/(64\cdot x^(4))`

`f''(x) = -(3)/(8\cdot x^(3))+(\ln (2\cdot x))/(4\cdot x^(3))`

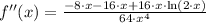
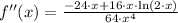
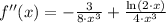
Now, let equalise the first derivative to zero and solve the resulting expression:

`(1-\ln(2\cdot x))/(8\cdot x^(2)) = 0`

`1-\ln(2\cdot x) = 0`

`\ln(2\cdot x) = 1`

`\ln 2 +\ln x = 1`

`\ln x = 1-\ln 2`

`x = e^(1-\ln 2)`

`x = (e)/(e^(\ln 2))`

`x = (e)/(2)`

`x \approx 1.359`

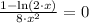
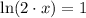
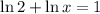
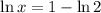
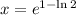
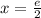
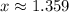
This result is evaluated at the second derivative expression:

`f''(1.359) =-(3)/(8\cdot (1.359)^(3))+(\ln [2\cdot (1.359)])/(4\cdot (1.359)^(3))`

`f''(1.359)\approx -0.050`

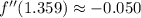
The critical value leads to a critical maximum and there are two intervals:

`(0, 1.359)` - Increasing

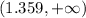`(1.359,+\infty )` - Decreasing

The graphic of the function is presented below as attachment.