Question

Show that 3 · 4^n + 51 is divisible by 3 and 9 for all positive integers n.

Answer 1

Explanation:

Hello, please consider the following.

`3\cdot 4^n+51=3\cdot 4^n+3\cdot 17=3(4^n+17)`

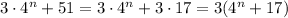
So this is divisible by 3.

Now, to prove that this is divisible by 9 = 3*3 we need to prove that

`4^n+17` is divisible by 3. We will prove it by induction.

Step 1 - for n = 1

4+17=21= 3*7 this is true

Step 2 - we assume this is true for k so
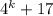`4^k+17` is divisible by 3

and we check what happens for k+1

`4^(k+1)+17=4\cdot 4^k+17=3\cdot 4^k + 4^k+17`

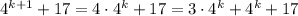
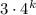`3\cdot 4^k` is divisible by 3 and

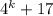`4^k+17` is divisible by 3, by induction hypothesis

So, the sum is divisible by 3.

Step 3 - Conclusion

We just prove that
`4^n+17` is divisible by 3 for all positive integers n.

Thanks