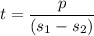Question

Given the equation p = s Subscript 1 Baseline t minus 2 Subscript 2 Baseline t, which equation is solved for t?

Answer 1

`t=(p)/((s_1-s_2))`

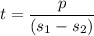
Explanation:

It is given that, p = s Subscript 1 Baseline t minus 2 Subscript 2 Baseline t. It can also written as :

`p=s_1t-s_2t`

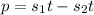
We need to solve the above equation for t.

Taking t common on RHS of the equation :

`p=(s_1-s_2)t`

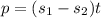
Now dividing both sides by
`(s_1-s_2)`, so,

`(p)/((s_1-s_2))=((s_1-s_2))/((s_1-s_2))t\\\\t=(p)/((s_1-s_2))`

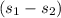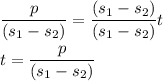
So, the equation for t is :
`t=(p)/((s_1-s_2))`