Answer:
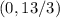
Explanation:
Since we know the slope and a point, we can use the point-slope form.
Recall that the point-slope form is:

Let m be 2/3 and let (-5,1) be x₁ and y₁. Therefore:
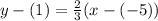
Simplify:
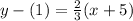
Distribute:
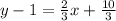
Add 1 to both sides. The left cancels. 1 is the same as 3/3:

Simplify:

This is in slope-intercept form. The coefficient is the slope and the y-intercept is the constant. Thus, the y-intercept is 13/3.