Question

A pure copper sphere has a radius of 0.935 in. How many copper atoms does it contain? [The volume of a sphere is (4>3)pr3 and the density of copper is 8.96 g>cm3.]

Answer 1

=4.8*10^-24atoms

Step-by-step explanation:

To find volume of a sphere

= 5.61*10^-5m³ x 8.96gx 100³m³

=502.7g

So we use density of copper to convert volume to mass so

1atm of cux( 1mol of cu/6.02Eatm of cu)*(63.54g of cu/1 mole of cu)

So = 1.055*10-22g cu

So

= 502.7/1.055*10-22g cu

=4.8*10^-24atoms

Answer 2

47.68 x 10²³ or 4.768 x 10²⁴ copper atoms

Step-by-step explanation:

Given:

Radius of the copper sphere (r) = 0.935 in

First convert the radius from inches to centimeters

1 in = 2.54cm

0.935in = 0.935 x 2.54cm = 2.3749cm

∴ r = 2.3749cm

Calculate the volume of the copper sphere as follows

Volume =
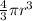`(4)/(3)\pi r^(3)` [substitute r = 2.3749cm and π = 22 / 7]

Volume =
`(4)/(3)((22)/(7) ) (2.3749)^(3)`

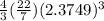
Volume = 56.108cm³

From the volume and given density, calculate the mass of the copper sphere

mass = density x volume [density = 8.96g/cm³]

mass = 8.96 x 56.108 = 502.73g

From known facts

1 mole of copper = 63.5g of copper = 6.022 x 10²³ copper atoms.

Then,

502.73 g of copper =
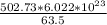`(502.73 * 6.022*10^(23))/(63.5)` = 47.68 x 10²³ copper atoms

Therefore, the sphere contains 47.68 x 10²³ copper atoms