Question

Radio waves of frequency 1.667 GHzGHz arrive at two telescopes that are connected by a computer to perform interferometry. One portion of the same wave front travels 1.260 mm farther than the other before the two signals are combined.

Required:
a. Will the two waves combine constructively or destructively?
b. Calculate the value of m for the path difference between the two signals

Answer 1

Step-by-step explanation:

The frequency of radio waves is 1.667 GHz

One portion of the same wave front travels 1.260 mm farther than the other before the two signals are combined.

There are two conditions for interference either constructive or destructive.

For constructive interference , the path difference is n times of wavelength and for destructive interference, the path difference is (n+1/2) times of wavelength

We can find wavelength in this case as follows :

`\lambda=(c)/(f)\\\\\lambda=(3* 10^8)/(1.667* 10^9)\\\\\lambda=0.1799\ m`

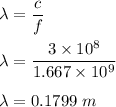
If we divide path difference by wavelength,

`(\delta)/(\lambda) =(1.26)/(0.1799)\\\\(\delta)/(\lambda) =7\\\\\delta =7\lambda`

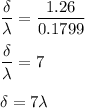
It means that the path difference is 7 times of the wavelength. it means the two waves combine constructively and the value of m for the path difference between the two signals is 7.