Answer: See below
Explanation:
A.
Let's split the integral into two parts, by the Sum Rule.
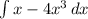 [split into 2 integrals]
[split into 2 integrals]
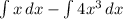 [solve integral for each part]
[solve integral for each part]
 [Remember, we need to add C for constant]
[Remember, we need to add C for constant]
-------------------------------------------------------------------------------------------------
B.
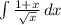 [expand into 2 integrals]
[expand into 2 integrals]
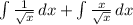 [simplify second integral]
[simplify second integral]
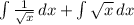 [solve integral for each part]
[solve integral for each part]
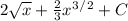
-------------------------------------------------------------------------------------------------
C.
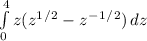 [distribute]
[distribute]
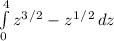 [split into 2 integrals]
[split into 2 integrals]
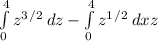 [solve integral for each part]
[solve integral for each part]
 [solve]
[solve]
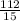
-------------------------------------------------------------------------------------------------
D. *Note: I can't put -1 for the interval, but know that the 1 on the bottom is supposed to be -1.
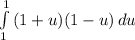 [expand]
[expand]
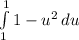 [split into 2 integrals]
[split into 2 integrals]
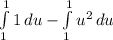 [solve integral for each part]
[solve integral for each part]
 [solve]
[solve]
