Question

The quadratic function g(x) has a vertex at
(-5, 0) and y-intercept of (0, -5). What is g(1)?

Answer 1

Answer: -7.2

Explanation:

The vertex form of quadratic equation :
`f (x) = m(x - a)^2 + b,` where (a,b) is the vertex.

Given: The quadratic function g(x) has a vertex at (-5, 0).

Put (a,b)= (-5,0) for function g(x), we get

`g(x)=m(x-(-5))^2+0\\\\\Rightarrow\ g(x)=m(x+5)^2`

Also, its y-intercept is (0, -5).

Put x= 0 and g(x) =-5

`-5=m(0+5)^2\\\\\Rightarrow\ -5=m(25)\\\\\Rightarrow\ m=(-5)/(25)\\\\\Rightarrow\ m=(-1)/(5)`

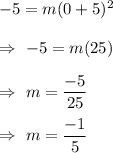
so,
`g(x)=-(1)/(5)(x+5)^2`

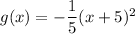
For g(1), put x=1

`g(1)=-(1)/(5)(1+5)^2\\\\=-(1)/(5)(6)^2=-(1)/(5)(36)\\\\=-7.2`

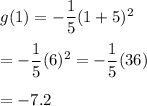
Hence, g(1) is -7.2.