Question

A rectangular garden is fenced on all sides with 128 feet of fencing. The garden is 4 feet longer than it is wide. Find the length and width of the garden.

Answer 1

Length = 34 and Width = 30

Explanation:

This is a simple system of equation that is mainly solved using substitution, so keep in mind the following key points:

• Rectangle's Perimeter is 128 ft.

• "the garden is 4 feet longer than it is wide," this simply means that the Length is 4 feet longer than the Width: which algebraically translates to
  `L = 4 + W`

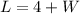
• Perimeter formula for Rectangle is
  `P = 2(L+W)`

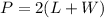
First, we find the Width by plugging the given values in the formula:

`128 = 2((4 + W) + W)\\128 = 8 +2W + 2W\\120 = 2W + 2W\\120 = 4W\\W = (120)/(4)\\\ W = 30`

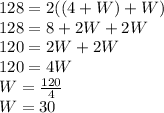
All we did here was substituting 4 + W for L to find the Width.

Now, to find the Length we plug in the value of W and solve for L:

`128 = 2(L + 30)\\(128)/(2) = (L + 30)\\64 = L + 30\\64 - 30 = L\\L = 34`

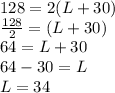
Tip: always sketch a rough geometrical diagram of word problems, it helps. (Refer to the diagram attached)

Keep working hard and good luck. <3