Question

The total combined volume of a glass, a jar, and a pitcher is 54 liters. If the jar has the same volume as 3 glasses and the pitcher has the same volume as 5 glasses, what is the volume of the glass?

Answer 1

`\Large \boxed{\sf 6 \ liters}`

Explanation:

Let the volume of the glass be x.

Let the volume of the jar be y.

Let the volume of the pitcher be z.

`\sf y = 3x \\\\z = 5x`

`\sf x + y + z = 54`

Substitution method.

`\sf x+3x+5x=54`

Combining like terms.

`\sf 9x = 54`

Dividing both sides by 9.

`\sf x = 6`

The volume of the glass is 6 liters.

Answer 2

6 liters.

Explanation:

So we have a glass, a jar, and a pitcher and together, they hold 54 liters.

We are told that the jar has the same volume as 3 glasses and the pitcher has the same volume as 5 glasses. In other words, let g denote glasses, j denote jars, and p denote pitchers:

`g+j+p=54`

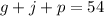
Since jar is the same as 3 glasses, this means that j=3g. And since the pitcher is the same as 5 glasses, this means that p=5g. Substitute:

`g+(3g)+(5g)=54`

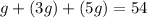
Combine like terms:

`9g=54`

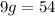
Divide both sides by 9. The left cancels:

`(9g)/9=54/9\\g=6`

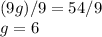
Therefore, the volume of the glass is 6 liters.