Question

SAT question, level of difficulty: HARD

-
-
The surface area, S, of a cylinder with a radius of 5 is defined by
`S = 2\pi(5^(2)) + 2\pi(5)h`, where h is the height of the cylinder. If the equation is rewritten in the form
`h = (S)/(x)-y`, where x and y are constants, what is the value of y ? (Surface Area
`= 2\pi rh+2\pi r^(2)`)
-
-
The answer is y = 5, but I can't figure out how, please help.

Answer 1

`S=2\pi (5^(2) )+2\pi (5)h\\h=(S)/(x) -y\\\\\S=2\pi (5^(2) )+2\pi (5)h\\S=2\pi (25)+10\pi h\\S= 50\pi +10\pi h\\10\pi h= S-50\pi \\h=(S-50\pi )/(10\pi ) \\h=(S)/(10\pi) - (50\pi )/(10\pi ) \\h=(S)/(x)-y = (S)/(10\pi) - 5\\y = 5`

This is a simplified version of ricchad's answer, all credit goes to that person.

Answer 2

y = 5

Step-by-step explanation:

S = 2π r² + 2π r h

let r = 5

let h = height of the cylinder

since the equation is re-written in the form h =
`(S)/(x) -y`

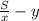
where x and y are constants.

what is the value of y?

S = (2π r²) + (2π r h) ------ plug in r = 5

S = (2π * 5²) + (2π * 5 * h)

S = (2π * 50) + (10π h)

S = 50π + 10π h

S - 50π = 10π h

S - 50π

h = -------------

10π

S 50π

h = ------ - ---------

10π 10π

S

h = ------ - 5

10π

therefore, the value of y = 5

remember the re-written equation h =
`(S)/(x) -y`

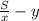
and x and y are constants.

x = 10π as constant

y = 5 as constant

hope it clears your mind.