Answers & Step-by-step explanation:
A square root is a number that when multiplied by itself, equals the number you were asked to square root. For example, 2 is the square root of 4, because 2 x 2 = 4.
There is a method in algebra to simplify square roots into smaller square roots, where one can be solved. To do this, we need to find a perfect square that can be multiplied with the other square root to equal the number you were asked to square root.
Now, let's solve your problems!
___________________________________________________________
5.
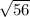 =
=

Now, we pull out the
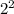 from under the radical, since we can square root this.
from under the radical, since we can square root this.
 is simply 2.
is simply 2.

Since we can't solve
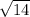 , this is the final answer that your assignment is wanting.
, this is the final answer that your assignment is wanting.
___________________________________________________________
6.
 =
=

Now, we pull out the
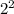 from under the radical, since we can square root this.
from under the radical, since we can square root this.
 is simply 2.
is simply 2.

Since we can't solve
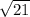 , this is the final answer.
, this is the final answer.
___________________________________________________________
7.
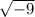 =
=
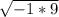
Now, since this the number is negative, we must separate the negative out. so
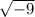 becomes
becomes
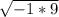 . Since the square root of 9 is 3, we can rewrite this as:
. Since the square root of 9 is 3, we can rewrite this as:
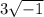
However, since
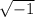 is imaginary, we must replace it with i. This also allows us to get rid of the square roots.
is imaginary, we must replace it with i. This also allows us to get rid of the square roots.
The answer becomes:
3i
___________________________________________________________
8.
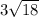
This one is already partly simplified. Notice how the 3 is not under the radical. The only thing we have to work with is the
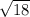 .
.
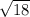 =
=
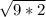
We can square root 9 to get 3.
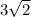
Now, we multiply back the 3 from earlier to get:
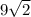
___________________________________________________________
9.
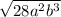
This one may look daunting because we have the variables, but we can break it down and solve it just as easily as the rest. The first step is to square root the a² term, the answer is a. We can rewrite this as:
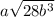
Next, we need to simplify
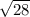 , just like we did with all the other problems.
, just like we did with all the other problems.
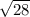 =
=
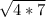
Since
 is 2, we can move it out of the radical.
is 2, we can move it out of the radical.
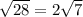
Since we can do anything with the
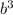 term, that stays under the radical.
term, that stays under the radical.
The answer is:

___________________________________________________________
I AM ALWAYS HAPPY TO HELP :)