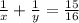Answer:
The sum of the reciprocal of two numbers are
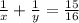
Explanation:
Step(i):-
Let x , y are two numbers
Given data the sum of two numbers = 15
x + y = 15 ...(i)
The product of two numbers = 16
x y = 16 ...(ii)
we know that
(x-y)² = (x + y)² - 4 x y
= (15)²- 4(16)
= 225 - 64
= 161
x-y = 12.68 ≅13 ...(iii)
Step(ii):-
We have
x + y = 15 ...(a)
x -y = 13 ...(b)
Solving (a) and (b)
2x = 27.68
x = 13.84
Substitute x = 13.84 in equation (i)
x + y = 15
13.84 + y = 15
y = 15 - 13.84
y = 1.16
Step(iii):-
The positive numbers are x = 13.84 and y = 1.16
The sum of the reciprocal of two numbers are

=
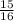
Conclusion:-
The sum of the reciprocal of two numbers are