Answer:
a
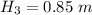
b

Step-by-step explanation:
From the question we are told that
The coefficient of resolution is
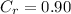
The initial height is
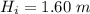
Considering question a
The number of times the ball bounced is
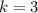
Generally the height attained after the 3rd bounce is mathematically represented as

=>
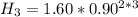
=>
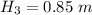
Considering question b
The mass is m = 48 g = 0.048 kg
Generally the amount of potential energy that was lost is mathematically represented as
![PE = mg [ H_i - H_3 ]](https://img.qammunity.org/2021/formulas/physics/college/l7wb9lvggi78k1td0pn3sif9890guuiv5c.png)
=>
![PE = 0.048 * 9.8 [1.60 - 0.85 ]](https://img.qammunity.org/2021/formulas/physics/college/e9liammx80166duua8ud4ihyp8hfex4jaq.png)
=>
