Question

A long, thin superconducting wire carrying a 17 A current passes through the center of a thin, 3.0-cm-diameter ring. A uniform electric field of increasing strength also passes through the ring, parallel to the wire. The magnetic field through the ring is zero.

a. At what rate is the electric field strength increasing?
b. is the electric field in the direction of the current or opposite to the current?

Answer 1

a

`(dE)/(dt) =- 2.72 *10^(15) \ N/C \cdot s`

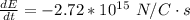
b

The direction of the electric field is opposite that of the current

Step-by-step explanation:

From the question we are told that

The current is
`I = 17\ A`

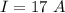
The diameter of the ring is
`d = 3.0 \ cm = 0.03 \ m`

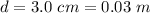
Generally the radius is mathematically represented as

`r = (d)/(2)`

`r = (0.03)/(2)`

`r = 0.015 \ m`

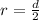
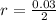
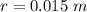
The cross-sectional area is mathematically represented as

`A = \pi r^2`

=>
`A = 3.142 * (0.015^2)`

=>
`A = 7.07 *10^(-4 ) \ m^ 2`

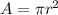
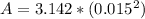
Generally according to ampere -Maxwell equation we have that

`\oint \= B \cdot \= ds = \mu_o I + \epsilon_o \mu _o( d \phi )/(dt )`

Now given that
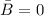`\= B = 0` it implies that

`\oint \= B \cdot \= ds = 0`

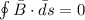
So

`\mu_o I + \epsilon_o \mu _o( d \phi )/(dt ) = 0`

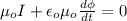
Where
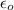`\epsilon _o` is the permittivity of free space with value
`\epsilon_o = 8.85*10^(-12 ) \ m^(-3) \cdot kg^(-1)\cdot s^4 \cdot A^2`

`\mu_o` is the permeability of free space with value

`\mu_o = 4\pi * 10^(-7) N/A^2`

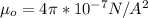
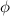`\phi` is magnetic flux which is mathematically represented as

`\phi = E * A`

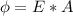
Where E is the electric field strength

So

`\mu_o I + \epsilon_o \mu _o ( d [EA] )/(dt ) = 0`

=>
`(dE)/(dt) =- (I)/(\epsilon_o * A )`

=>
`(dE)/(dt) =- (17)/(8.85*10^(-12) * 7.07*10^(-4) )`

=>
`(dE)/(dt) =- 2.72 *10^(15) \ N/C \cdot s`

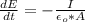
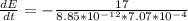
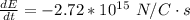
The negative sign shows that the direction of the electric field is opposite that of the current