Question

If the mean of: 2x,x,(2x−11),(x−3) is equal 4, then the value of x is:

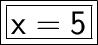
Answer 1

`\boxed{ \bold{ \huge{ \boxed{ \sf{x = 5}}}}}`

Explanation:

Given data : 2x , x , 2x - 11 , x - 3

Mean = 4

N ( number of items ) = 4

Σx = 2x + x + 2x - 11 + x - 3

To find : value of x

Finding the value of x

We know that ,

Mean =
`\sf{ (Σx)/(n) }`

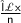
plug the values

⇒
`\sf{4 = (2x + x + 2x - 11 + x - 3)/(4) }`

Collect like terms

⇒
`\sf{4 = (6x - 14)/(4 )}`

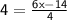
Apply cross product property

⇒
`\sf{6x - 14 = 16}`

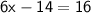
Move 14 to right hand side and change it's sign

⇒
`\sf{6x = 16 + 14}`

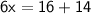
Add the numbers

⇒
`\sf{6x = 30}`

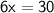
Divide both sides of the equation by 6

⇒
`\sf{ (6x)/(6) = (30)/(6) }`

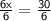
Calculate

⇒
`\sf{x = 5}`

Hope I helped!!

Best regards!!!