Answer:
Step-by-step explanation:
force constant of spring k = force / extension
= 35.6 / 0.5
k = 71.2 N / m
angular frequency ω of oscillation by spring mass system
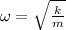
where m is mass of the body attached with spring
Putting the values
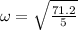
ω = 3.77 radian / s
The oscillation of the mass will be like SHM having amplitude of 0.5 m and angular frequency of 3.77 radian /s . Initial phase will be π / 2
so the equation for displacement from equilibrium position that is middle point can be given as follows
x = .5 sin ( ω t + π / 2 )
= 0.5 cos ω t
= 0.5 cos 3.77 t .
x = 0.5 cos 3.77 t .