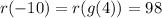Answer:
98
Explanation:
So we have the two functions:
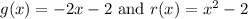
And we want to find the value of r(g(4)).
To do so, first find the value of g(4):

Multiply:
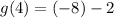
Subtract:
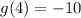
Now, substitute this into r(g(4)):
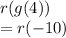
And substitute this value into r(x):
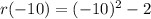
Square:
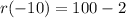
Subtract:
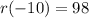
Therefore: