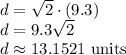Answer:
Approximately 13.1521 units.
Explanation:
To find the diagonal, we can use the Pythagorean Theorem.
Since the figure is a square, all four sides are equivalent. A square also has four right angles. Therefore, we can use the Pythagorean Theorem to find the diagonal d. Therefore:

Substitute 9.3 for a and b, and let c equal d:
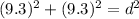
Instead of squaring, add the like-terms:
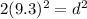
Take the square root of both sides:
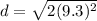
Expand:
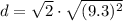
The right cancels: