Answer:
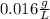
Step-by-step explanation:
Hello,
In this case, the dissociation of calcium fluoride is:
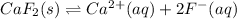
And the equilibrium expression is:
![Ksp=[Ca^(2+)][F^-]^2](https://img.qammunity.org/2021/formulas/chemistry/college/zzmc07yep1karrfpe89mfohuprmzyybi82.png)
Which is useful to compute the molar solubility, symbolized by
 as the reaction extent:
as the reaction extent:
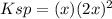
In such a way, since the solubility product of calcium fluoride at 25 °C is 3.45x10⁻¹¹, the molar solubility is found to be:
![3.45x10^(-11)=(x)(2x)^2\\\\x=\sqrt[3]{(3.45x10^(-11))/(4) }\\ \\x=2.05x10^(-4)M=2.05x10^(-4)(molCaF_2)/(L)](https://img.qammunity.org/2021/formulas/chemistry/high-school/oxdgw389oo9ervjkdq6yekdv0rqt6ba6ld.png)
And the solubility, considering its molar mass 78.08 g/mol is:
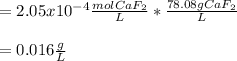
Regards.