Answer:
210.33 cm^2
Explanation:
We know that 6 equilateral triangles makes one hexagon.
Also, an equilateral triangle has all its sides equal.
If the tile of each side of the triangular tile measure 9 cm, then the height of the triangular tiles can be gotten using Pythagoras's Theorem.
The triangle formed by each tile can be split along its height, into two right angle triangles with base (adjacent) 4.5 cm and slant side (hypotenuse) of 9 cm. The height (opposite) is calculated as,
From Pythagoras's theorem,

substituting, we have
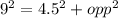
81 = 20.25 +

 = 81 - 20.25 = 60.75
= 81 - 20.25 = 60.75
opp =
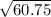 = 7.79 cm this is the height of the right angle triangle, and also the height of the equilateral triangular tiles.
= 7.79 cm this is the height of the right angle triangle, and also the height of the equilateral triangular tiles.
The area of a triangle =
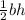
where b is the base = 9 cm
h is the height = 7.79 cm
substituting, we have
area =
 x 9 x 7.79 = 35.055 cm^2
x 9 x 7.79 = 35.055 cm^2
Area of the hexagon that will be formed = 6 x area of the triangular tiles
==> 6 x 35.055 cm^2 = 210.33 cm^2