Answer:
The first derivative of
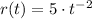 (r(t)=5*t^{-2}) with respect to t is
(r(t)=5*t^{-2}) with respect to t is
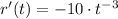 (r'(t) = -10*t^{-3}).
(r'(t) = -10*t^{-3}).
Explanation:
Let be
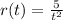 , which can be rewritten as
, which can be rewritten as
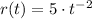 . The rule of differentiation for a potential function multiplied by a constant is:
. The rule of differentiation for a potential function multiplied by a constant is:
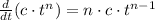 ,
,
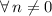
Then,
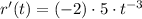
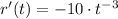 (r'(t) = -10*t^{-3})
(r'(t) = -10*t^{-3})
The first derivative of
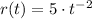 (r(t)=5*t^{-2}) with respect to t is
(r(t)=5*t^{-2}) with respect to t is
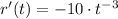 (r'(t) = -10*t^{-3}).
(r'(t) = -10*t^{-3}).