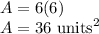Question

Rectangle PQRS has vertices at P-2,5), Q44,5), R(4, -1), and S(-2,-1). What is the area, in square units, of rectangle PQRS? 21 b 35 36 40​

Answer 1

36 square units.

C

Explanation:

Please refer to the provided graph.

So we want to figure out the area of the rectangle. To do so, we simply need to figure out the base and height of the rectangle. We can then multiply them together to acquire the area.

From the graph, we can see that if we find the length of SR and SP, we can use the the base and height, respectively.

Now, we just have to find there lengths. Technically, we could just count. However, I'm going to use the distance formula.

the distance formula is:

`d=√((x_2-x_1)^2+(y_2-y_1)^2)`

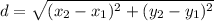
Find the length of SR:

Point S is (-2,-1) while Point R is (4,-1). Let (-2,-1) be x₁ and y₁ and (4,-1) be x₂ and y₂. Therefore:

`d=√((4-(-2))^2+(-1-(-1))^2)\\d=√((6^2)+(0)^2) \\d=√(36)=6`

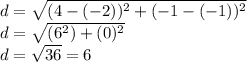
So, SR is 6.

Now, find the length of SP:

Point S is (-2,-1) while Point P is (-2,5). Let Let (-2,-1) be x₁ and y₁ and (-2,5) be x₂ and y₂. Therefore:

`d=√((-2-(-2))^2+(5-(-1))^2)\\d=√(0^2+6^2)\\ d=√(36)=6`

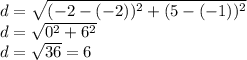
Therefore, SP is also 6.

Now, the area for a rectangle is:

`A=bh`

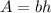
Plug in 6 for the base and 6 for the height:

`A=6(6)\\A=36\text{ units}^2`